Research

Floquet topological states

Topology is one of the key concepts in modern condensed matter physics. It was believed that "topology is robust against perturbation" and is something that is fixed when a material is synthesized. We found a way to change the topology of materials dynamically. In 2009, we found a theoretical model in which the Berry curvature, Chern number, and the chiral edge state could be controlled or induced by external time-periodic fields such as a laser. The key idea is that electrons become photo-dressed. They are described by Floquet states, a temporal analog of Bloch states, and the topology of the quasi-energy bands can be changed as a function of the laser intensity, polarization, and frequency.

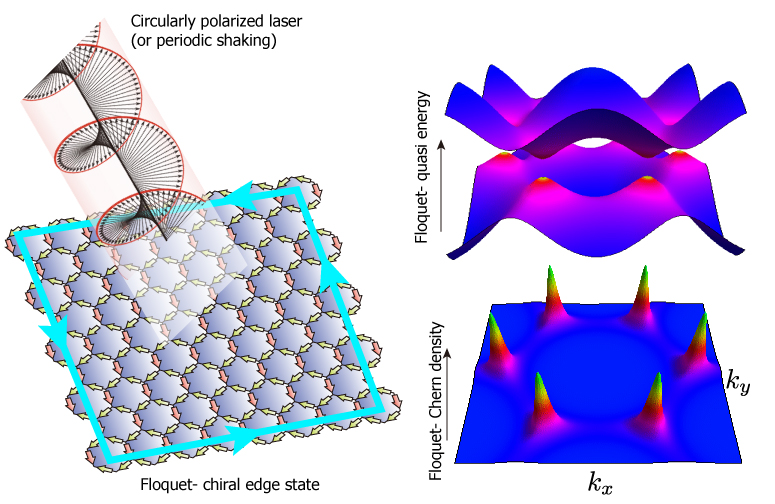
Figure: (Left) Honeycomb lattice in circularly polarized laser becomes a Floquet Chern insulator.
(Right) The Floquet quasi-energy in the high frequency limit shows a gap opening at the Dirac points. This leads to the emergence of the Floquet Berry curvature.

Floquet Engineering of Relativistic Electrons

Preprint: [Oka, arXiv:2407.21458]
This study investigates the changes in electronic states caused by waves V(Qx−ωt) propagating through quantum materials. These waves include laser light, polaritons, acoustic waves, and sliding density waves, with a wide range of speeds. Since these waves are periodic in both space and time, electrons in the materials form Floquet-Bloch states under their influence. Using three-dimensional (3D) Dirac electrons in circularly polarized laser waves as a case study, the research examines band engineering induced by these waves.
The waves are classified into three categories—temporal, light-like, and spatial waves—based on their speed, and distinctive electronic states are identified for each case. Additionally, the study demonstrates phase transitions in electronic bands and reveals the emergence of Floquet Weyl bands, which can potentially transition to Type-II bands near the phase transition. The effect of Floquet band engineering is shown to be enhanced by Lorentz contraction, which is maximized when the wave speed approaches the Fermi velocity. Furthermore, the geometric nature of response currents is discussed.

Inverse Spin Hall Effect in Nonequilibrium Dirac Systems

Preprint: [Teh, Numasawa, Okumura, Oka, arXiv:2409.09025]
This study analyzes Dirac fermions in the presence of a space-dependent chiral gauge field and thermodynamic gradients, establishing a connection to the inverse spin Hall effect. The chiral gauge field induces a chiral magnetic field, resulting in surface Fermi arc states and a chiral Landau level state, which, although delocalized in the bulk, is shown to be more robust against impurities.
By applying chemical potential and temperature gradients, nonzero charge currents are generated, with each gradient leading to distinct Fermi level dependencies. These properties have been observed in recent experiments. Unlike the conventional mixed axial-gravitational anomaly, the currents demonstrated in this study require a noncollinear chiral magnetic field and thermodynamic gradients.
Additionally, the study derives low-energy transport formulas and highlights the importance of carefully handling the ultraviolet cutoff for understanding lattice calculations.